
Theodore Kim, Fernando de Goes, Hayley Iben
Abstract:
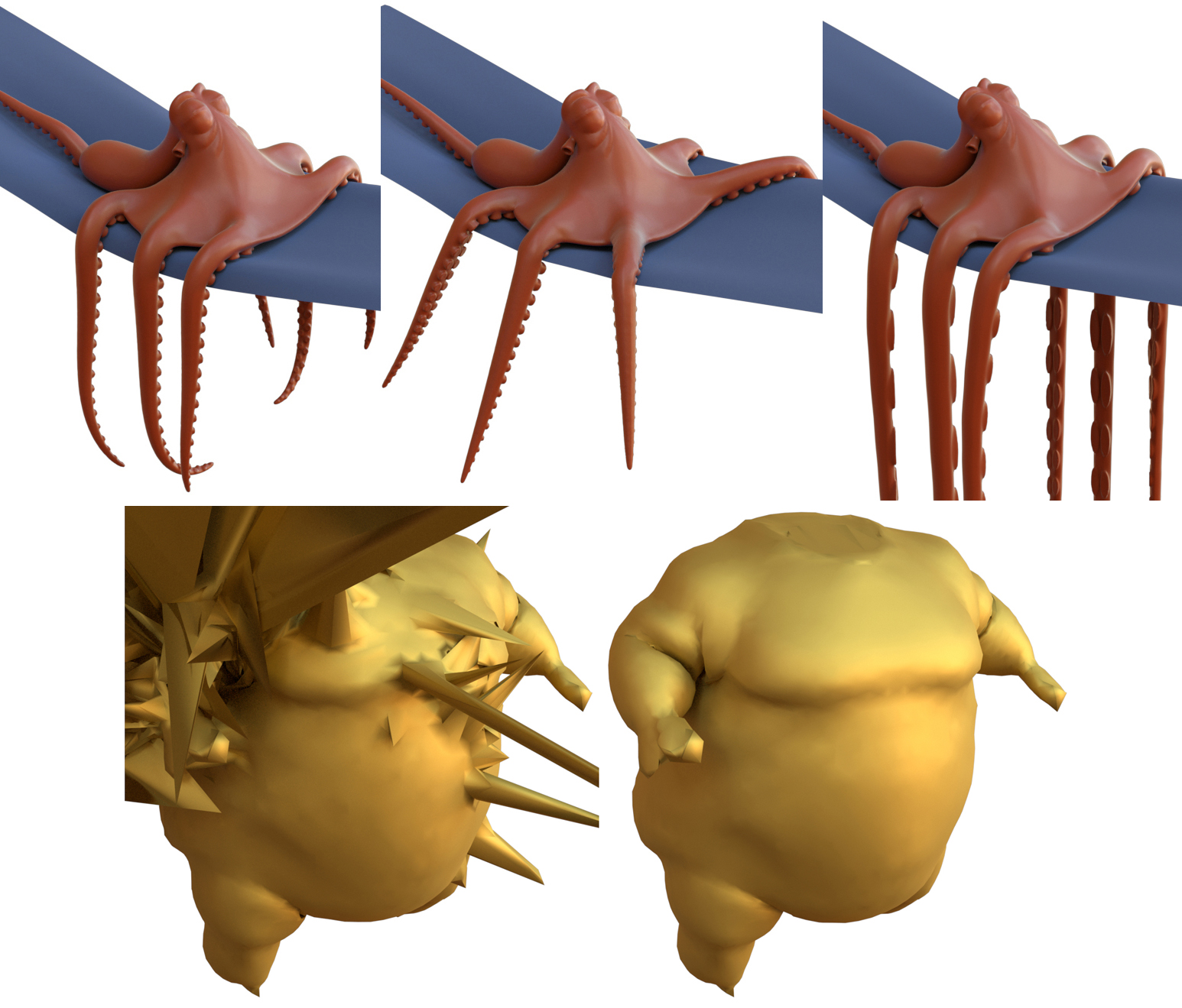
We present an analysis of anisotropic hyperelasticity, specifically transverse isotropy, that obtains closed-form expressions for the eigendecompositions of many common energies. We then use these to build fast and concise Newton implementations. We leverage our analysis in two separate applications. First, we show that existing anisotropic energies are not inversion-safe, and contain spurious stable states under large deformation. We then propose a new anisotropic strain invariant that enables the formulation of a novel, robust, and inversion-safe energy. The new energy fits completely within our analysis, so closed-form expressions are obtained for its eigensystem as well. Secondly, we use our analysis to badly-conditioned finite elements. Using this method, we can robustly simulate large deformations even when a mesh contains degenerate, zero-volume elements. We accomplish this by swapping the badly-behaved isotropic direction with a well-behaved anisotropic term. We validate our approach on a variety of examples.
Paper (PDF)
Additional materials: [Anisotropy2019_supplement.pdf], [matlab.zip]
Video

